Energy

Global energy demand is increasing rapidly. According to Statista, it will reach 760 exajoules in 2050, this is the equivalent of 155 billion barrels of oil and a 21% increase from 2019.  Sources of power are multiplying as people are moving to smart homes using solar cells and their own wind turbines. New types of vehicles are emerging, thus new ways of charging them. All of this must happen while decreasing carbon emissions.  

In other terms, we are seeing an exponential increase in the quantity of data from the energy sector, while having more and more stringent conditions on the routing of electricity. These are the typical types of problems that Quantum Computing is suited for, and traditional computers cannot tackle. 

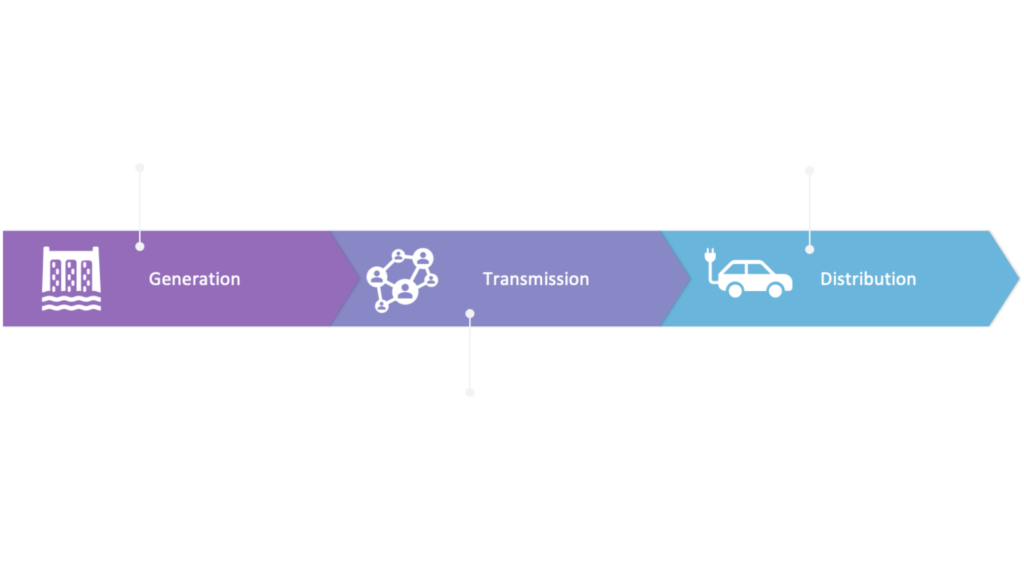
Quantum Computing can have an impact on the entire supply chain of energy: 

  • Generation: Simulating the combustion reactions in engines or the generation of wind energy with high precision goes beyond the capacity of today’s supercomputers. In a similar vein, computing the structure of gigantic power plants to make them safe is extremely computationally intensive (see detailed use-cases below).  
  • Transmission: Optimising the planning and routing of electricity, allocating scarce resources, and making smart grids more secure and efficient can greatly enhance the transmission of energy. 
  • Distribution: The distribution stage of the energy supply chain can be improved by the detection of faults using Quantum Machine Learning or designing new batteries with enhanced quantum chemistry techniques.  

In short, Quantum Computing will have a transversal impact on the Energy sector and will be a key competitive advantage in the years to come. 

Quandela’s Use Cases 

Clients: EDF​ 

Challenge (What): Solve systems of differential equations on a quantum computer.​ 

Application (Why): Quandela and EDF have developed a quantum algorithm which models the mechanical structure of power plants, with the outlook to improve maintenance scheduling for safer structures.​ 

Methodology (How): We use a variational quantum algorithm to solve a set of partial differential equations. The set of differential equations is first converted into a linear system of equations, whose solution is represented by the minimum energy of a quantum observable. This solution may lead to an exponential speed-up compared to the classical analog. 

READ CASE STORY

Quantum Computing Use Cases Explained: Energy Infographic

Who are we

Quandela is a European start up that provides photonic quantum computers that are modular, scalable, energy-efficient and accessible both on the cloud and on premise. Our team specialises in the development of both software and hardware solutions for a variety of quantum applications. We offer a wide range of services, from developing the most efficient and brightest single photons sources, to creating algorithms for quantum computers and providing cloud quantum computing solutions. 

+

Join our team

As an applicant at Quandela, you will join a dynamic and international team of scientists, engineers, technologists, and business professionals. You will have the opportunity to work on cutting-edge technologies and contribute to the development of innovative solutions that will have a global impact. We are looking for passionate and ambitious people who are eager to learn and grow with us. 

APPLY NOW

Who trusts us

At Quandela, we are committed to providing end-users with the best quantum computing experience. Our team specialises in the development of both software and hardware solutions for a variety of quantum applications and provides you with the most reliable and cost-effective solutions. Our goal is to make quantum computing accessible and easy to use for everyone. Our technology is backed by the world's leading industry partners, investors, and funding agencies – join us and unlock the potential of quantum computing. 

+

Ask for solutions

Take your business to the next level with Quantum Computing. We help companies solve their business challenges with quantum computing. Learn how to leverage our quantum technology in your product or service now!